Formula Quick Look

The following page is a list of formulas and very brief explanations.

Density is the ratio of a substance's mass to its volume and can be expressed mathematically as

D=M/V

where D is density, M is mass, and V is volume.

Example:

What is the density of an object having a mass of 8 kg and a volume of 2 cubic meters?
D = M/V
D = 8/2
D = 4 kg/m³

Temperature:
In science, we will use Celsius or Kelvin temperature scales to describe temperature.

To convert between Celsius and Kelvin:

Kelvin = Celsius + 273.15
Celsius = Kelvin - 273.15

To convert between Celsius and Fahrenheit:

Fahrenheit = Celsius * (9/5) + 32
Celsius = (Fahrenheit - 32) * 5/9

Gas Laws:

Charles's Law
The volume of a gas is directly proportional to its temperature in kelvins if the pressure and number of molecules are constant.

V1T1=V2T2  

Boyle's Law
The volume of a gas is inversely proportional to its pressure if the temperature and the number of molecules are constant.

P1V1=P2T2

Combined Gas Law
Pressure is inversely proportional to volume, or higher volume equals lower pressure. Pressure is directly proportional to temperature, or higher temperature equals higher pressure.

(P1V1)/T1=(P2V2)/T2 

Example:

If a sample of gas initially has a pressure of 2 atm, a volume of 3 liters, and a temperature of 300 K, what would its final volume be if the pressure changed to 1.5 atm and the temperature changed to 290 K? 

(P1V1)/T1=(P2V2)/T2 (2 • 3)/300 = (1.5 • V)/290
6/300 = 1.5 V/290
290 • (6/300) = 1.5 V
5.8 = 1.5 V
5.8/1.5 = V
3.87 l = V

Ideal Gas Law

When the number of molecules are included in calculations, the following formula can be used:

PV = nRT

This law can be converted into the following form which will allow memorization of only 1 formula for gas law problems:

P1V1     P2V2
_____ = _____
n1T1       n2T2 

Motion:

Finding final velocity:

vf = vi + at

where vf is final velocity, vi is initial velocity, a is acceleration, and t is elapsed time.

Example:

An object is moving at a rate of 3 m/s and accelerates at a rate of 2 (m/s)/s for 5 seconds. What is its final velocity? 

vf = vi + at
vf = 3 + 2 • 5
vf = 3 + 10
vf = 13 m/s

Finding average velocity:

v(ave) = (vf+vi)/2

where v(ave) is average velocity, vf is final velocity, and vi is initial velocity. Also:

v(ave) = ((vi + at) + vi)/2

Finding final position (final distance from a reference point):

df = di + vit + 1/2at²         

where df is the final, total displacement… 

di is the initial displacement. (How far from whatever point of reference is the object when the thing starts accelerating?)…

 vi is the initial velocity of the object at the beginning of the acceleration.

t is the elapsed time from the beginning of the acceleration until the end of the period being observed. 

(vit accounts for the motion of the object based on its starting velocity. It keeps covering distance at the initial rate, and additionally, it accelerates and covers more distance.) 

a is the acceleration and t is elapsed time.

Example:

An object begins 10 meters from a mark on a track with an initial velocity of 3 m/s. If it accelerates at a rate of 5 (m/s)/s for 4 seconds, how far from the mark does it end up? 

df = di + vit + 1/2at² 

df = 10 + 3(4) + 1/2(5)(4)^2
df = 10 + 12 + 1/2 (5) (16)
df = 10 + 12 + 40
df = 62 m

Force:

Where F is force, a is acceleration, and m is mass, then:

F = ma

Force of Friction—friction always opposes forces that are moving (or trying to move) an object.

Ff = Fnμ

where  Ff  is the force of friction,  Fn  is the normal force, and  μ is the coefficient of friction (a number that is looked up).

The normal force is the portion of weight that is perpendicular to the surface. For a flat surface (the angle between the surface and the horizon is zero, θ = 0):

Fn = mg

where m is mass and g is acceleration due to gravity (9.8 (m/s/)s on earth) 

Work and Energy:

Work is found by

W = Fd

where W is work, F is force applied (not net force!), and d is displacement/distance.

Kinetic energy (KE) is found with this equation:

KE = 1/2 mv²

where KE is kinetic energy, m is mass and v is velocity.


The potential gravitational energy can be found with this equation:

PE = mgh

where PE is potential gravitational energy, m is mass, g is acceleration due to gravity, and h is height.

On earth, acceleration due to gravity is 9.8 (m/s)/s


The amount of elastic potential energy is determined by how hard it is to compress or stretch something and how far it is stretched or compressed.

The equation to find this is:

PE = 1/2kd²

where PE is potential elastic energy, k is a constant specific to a particular stretchy thing (spring, rubber band, etc.) and d is the distance that it is stretched or compressed (sometimes x is used instead of d, as in the illustration)



Heat/Energy Transfer

Now to find heat, we can use the formula:

Q = mcΔT

Where Q is heat or thermal energy, m is mass, c is a number called specific heat that you either look up or calculate, and ΔT is the change in temperature.

EXAMPLES:

How much heat is absorbed by 200 grams of water that starts at 25C and ends up at 30C, given that the specific heat of water is 4.2 J/g°C?

Q = mcΔT

Q = 200•4.2•(30-25)

Q = 200•4.2•5

Q = 4200 J

What is the specific heat of a metal that has a mass of 50 grams and changes temperature from 100C to 30C and gives off 4200 J of thermal energy?

Q = mcΔT

4200 = 50•c•(100-30)

4200 = 50•70•c

4200 = 3500c

4200/3500 = c

1.2 = c

---

VARIOUS EXAMPLES

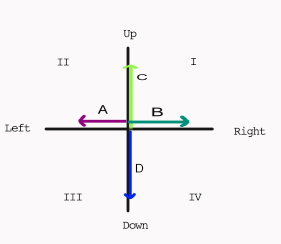
Given the following information, find the work done on a 6.5 kg object after 4 seconds:

A = 16 N
B = 4 N
C = 14 N
D = 9 N

 STEP 1: Find Net Force by resolving the UpDown forces, resolving the LeftRight forces, and then using the Pythagorean Theorem:


F(net)² = UpDown² + LeftRight²

F(net)² = (16 - 4)² +(14 - 9)²
F(net)² = (12)² + (5)²
F(net)² = 144 + 25
F(net)² = 169
F(net) = 13 N

STEP 2: Find Acceleration where the force is the net force on the object:

F = ma
13 = 6.5a
13/6.5 = a
2 (m/s)/s  =  a

STEP 3: Find the distance through which the force acted due to the net force.

df = 1/2at²
df = 1/2 • 2 • 4²
df = 16 m

STEP 4: Find the work done by the net force through the calculated distance.

W = Fd
W = 13 • 16
W = 208 J




To find the final velocity in the above:

Do Step 1 above.
Do Step 2 above.

STEP 3:

Vf = at
Vf = 2 • 4
Vf = 8 m/s


To find final kinetic energy, first find the final velocity (above), and then:

KE = 1/2mv²
KE = 1/2•6.5•8²
KE = 1/2 • 6.5 • 64
KE = 208 J